

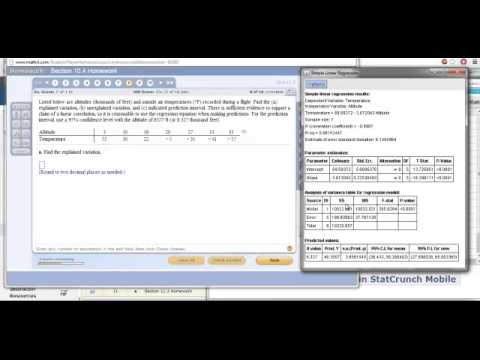
However, the z value (also called z score) and z table can be used to get the exact probability for any score.1 Draft IRPSummary:In the Independent Research Project (IRP), students conduct an analysis project using SAS primarily and Excel as needed to analyze real-world data.In the first assignment (IRP Research Question and Data Set), you identified the data set you wanted to use, developed a research question, and identified a statistical question that could be answered analytically.In this assignment, you will create and submit a business memo (template attached) that clearly communicates your analysis of the data set you identified in the previous assignment. NOTICE: These examples use the Empirical Rule to Estimate the Probability. To score ABOVE 88 there is only a 2.5% chance. Here, 88 is two deviations above the mean. Using the Empirical Rule, we can see that about 14% + 34% + 34% + 14% of scores are BETWEEN 74 and 88 and to there is a 95% chance that a score will be between 74 and 88. Here, 74 is two deviation below the mean and 88 is two deviations above the mean. So there is a 34% + 14% = 48% chance that a student will score between 81 and 74.Į) Probability that a score is between 74 and 88? Using the Empirical Rule, we can see that about 34% + 14% of scores are BETWEEN the mean and the second deviation below it. So, a score of 74 is 81 – 3.5 – 3.5 = 74 or TWO deviations below the mean. Why? Because each deviation in this question is “3.5” points. Next, the score of 74 is a two standard deviations BELOW the mean. Here, 81 is the mean, so we know that 50% of the class is below this point.
So there is 34% chance that a student will score between 81 and 84.5.ĭ) Probability that a score is between 81 (the mean) and 74? Using the Empirical Rule, we can see that about 34% of scores are BETWEEN the mean and the first deviation. So, a score of 84.5 is 81 + 3.5 or one deviation above the mean. Next, the score of 84.5 is a one standard deviation above the mean. The answer here is 50%Ĭ) Probability that a score is between 81 (the mean) and 84.5? Therefore, 50% of students are expected to score above this value and 50% below. In this example, the mean of the dataset (the average score) is 81.
Using this information, estimate the percentage of students who will get the following scores using the Empirical Rule (also called the 95 – 68 – 34 Rule and the 50 – 34 – 14 Rule): This dataset is normally distributed with a mean of 81 and a std dev of 3.5.
Suppose a teacher has collected all the final exam scores for all statistics classes she has ever taught.


 0 kommentar(er)
0 kommentar(er)
